The Pythagorean Experiment
A reader whom I somewhat insulted wrote me a rather nice letter in return, and, more importantly, asked about the kind of philosophical questions which delight me more than wine. I would answer the questions, except that his questions provoke more questions in me than answers. So I will lay these queries out for him or anyone else who cares to comment to answer.
For the sake of simplicity, I will not put all his questions in one piece, lest some thread the discussion be lost.
Also, I mean not to answer the questions in the order asked, but will answer the one that interests me most first. Let’s start in the middle!
Question Seven: How is the Pythagorean Theorem non-empirical? I can test it with a ruler and a protractor. I assume it’s true and all my math works out and the bridges I build with it don’t fall down, so I can feel confident in it’s factuality.
Webster’s defines “Empirical” to mean capable of being verified or disproved by observation or experiment. However, among philosophers, the word is a term of art with a more exact meaning: An empirical truth is a truth the senses (or logical deductions from them) have some tendency to prove or disprove.
Hence, an empirical truth is dependent on sense information, which means, a truth which is true only when and where the senses (or logical deductions from them) confirm it, and which relies on no other basis but the senses (or logical deductions from them) for proof of their truth.
This is in contrast with a rational truth. A rational truth is a truth deduced by logic from first principles, and, if the first principles are true, and the reasoning valid, the conclusion must be true. Hence, a rational truth is dependent on the truth of the first principles on which it rests, and on nothing else. Since rational truth depends on nothing else, it is true under all times, places, and conditions, no matter what the senses says or seem to say.
That is the definition used by all philosophers since the dawn of the discipline of philosophy. We cannot substitute another definition without running the risk of deception or confusion.
A thumbnail way to distinguish the two is by the imagination. If one can imagine conditions under which the conclusion is not true, then the conclusion is a conditional.
It is an empirical truth apple trees do not talk. Much evidence confirms it. However, if flown to Oz on a tornado, we might well encounter trees that grew apples, talked, and tossed their fruit in anger at little girls. While the talking apple tree of Oz is impossible in the sense that it cannot fit into the world as we know it, it is not impossible in the logical sense, that is, it does not violate the law of identity.
On the other hand, if the talking apple tree throws two apples with one limb and two with the other, then he has thrown four apples, and that conclusion is as rigid and inescapable in Oz as in Kansas, and nothing can be done to escape it.
There is no “Fiveland” where twice two is five, and it cannot be imagined, nor can logical deductions spring from the conclusion that twice two is five without contradiction other deductions equally as valid springing from the same conclusion.
Empirical conclusions are conditional upon empirical evidence of the senses. If no one can imagine conditions under which the conclusion is untrue, the conclusion is true absolutely, for then the conclusion depends on first principles and logical deductions from them.
That being said, let us engage in a bit of a thought experiment, or hypothetical, to see if a world can exist where the Pythagorean Theorem cannot be demonstrated rationally, that is, it is not a conclusion based on first principles, but can be demonstrated empirically, that is, by means of the sense impressions and logical deductions from them.
Suppose we have two men, Pythagoras and Empirico.
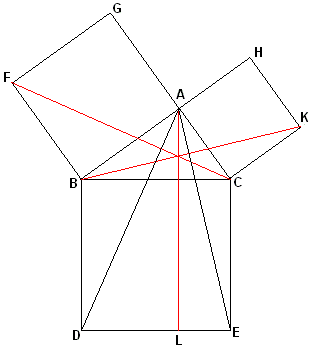
Pythagoras offers you the following argument. It is a little difficult to follow without a diagram: “In right-angled triangles the square on the hypotenuse equals the sum of the squares on the legs.
“Assume for the sake of argument that any triangles constructed on the same base and under the same parallel as a parallelogram has half the area. Assume two triangles having an equal angle adjacent legs equal are congruent.
“Let ABC be a right-angled triangle having the angle BAC right. I say that the square on BC equals the sum of the squares on BA and AC.
“For let be the square BDEC on BC, and the squares GB and HC on BA and AC.
“Since the angle DBC equals the angle FBA, for each is right, add the angle ABC to each, therefore the whole angle DBA equals the whole angle FBC.
“Since DB equals BC, and FB equals BA, the two sides AB and BD equal the two sides FB and BC respectively, and the angle ABD equals the angle FBC, therefore the base AD equals the base FC, and the triangle ABD equals the triangle FBC.
“Now the parallelogram BL is double the triangle ABD, for they have the same base BD and are in the same parallels BD and AL. And the square GB is double the triangle FBC, for they again have the same base FB and are in the same parallels FB and GC.
“Therefore the parallelogram BL also equals the square GB.
“Similarly, if AE and BK are joined, the parallelogram CL can also be proved equal to the square HC. Therefore the whole square BDEC equals the sum of the two squares GB and HC.”
Therefore the square on BC equals the sum of the squares on BA and AC. Which was what was to be proved.”
Of course, if you have an exacting and rigorous mind, you may ask for additional proof to show that the lines BH and GC are indeed straight lines, and ask for proof that triangles built on the same base and under the same height as a parallelogram are half its area. And you certainly need a proof concerning the congruence of triangles if a side an angle and an adjacent side are equal in both.
Even you are even more skeptical and rigorous, you will demand every step in every proof until you come to the first principles which cannot further be reduced to any underlying principles.
He can define his terms. For example, a point is that which has no part, a line is a length without breadth, a straight line is a line all points lying evenly on itself, and a triangle is a figure compose of three straight lines. A right triangle is one where one angle is formed by a perpendicular. And so on.
He can state certain common notions true not only in his discipline but in many others, namely, that things equal to the same are equal to each another; if equals be added to equals, the wholes are equal; if equals be subtracted from equals, the remainders are equal; Things which coincide with one another are equal to one another; The whole is greater than the part.
But there are certain postulates which you must grant him, if you wish to study his discipline: To draw a straight line from any point to any point; To produce a straight line continuously from a straight line; to describe a circle with any center and distance; that all right angles are equal to one another; that parallel line neither converge nor diverge. (There is an offshoot of his discipline called noneuclidean geometry which draws out the implications of accepting all postulates but the last, which is equally as rigorous, albeit less easy to depict visually.)
Please note that after a careful study of all his arguments, he has not once, either directly or by implication, asked you to examine anything open to any sense impression. He has made no measurements, pulled out no protractor, did not specify a date or place where his conclusions were true, nor did he specify under what conditions his conclusion was true and when it was not true.
Please note that the entities of which he speaks do not exist in the world of the sense impressions. There are very thin threads, but no length without breadth. There are dots and jots and tittles, but no entities which have location but lack all extension. There are triangular objects, physical things that look something like triangles and which remind us of triangles, but there are no triangles. We have never seen one. They cannot be seen.
Empirico offers you the following argument: “On or about the anniversary of this day a year from now, I shall draw three right triangles. One shall be a line of drool I will spit from my mouth onto a china plate. The second shall be drawn in the sand below the tideline with my finger. The third shall be drawn in a trail of puce colored smoke behind my cropdusting plane at ten thousand feet, inside a storm cloud.
“Then I will draw nine squares, each one on the sides of each of the triangles of spit and sand and puce smoke. The square on the hypotenuse of the smoke triangle will be equal in area to the squares on the two remaining sides but this will not be true of the spit and the sand triangle, due to the different conditions of cloud and altitude versus china plates and spit and sand and strand.
“Half a billion years ago, in a galaxy in the Corona Borealis Supercluster of Galaxies one billion lightyears hence, a race highly advanced above our own maneuvered three stars into the points of a right triangle, and connected those stars by a trail of fine particles of ice and dust plucked from nearby nebulae. Likewise, the square built out of additional nebular smoke on the hypotenuse of that stellar triangle did not have an area equal to the squares build on the remaining sides for several million years, but, later, when conditions changed, it did.
“Therefore, I urge you to pay not the least attention to what Pythagoras is saying. He has not offered you a single real triangle to examine, nor has he brought out his protractor, nor paced off the distance of any triangle you can see with your eye nor touch with your hand.”
Now, consider the fact that, if Empirico’ statements about time and distance are correct, you will not see any of the triangular objects he is discussing, not the triangle of sand, nor smoke, nor spit, nor stars, until any where between a year from now to half a billion years from now.
So you yourself cannot have any empirical knowledge of the properties of this triangles, not yet.
Also, the viewing conditions of all the triangles involved is doubtful, even if you did wait, since none of these substances form very crisp lines, and factors such as wind or wave or interstellar motions might easily disturb the arrangements.
Also, it is nearly impossible to get a protractor of the right size and maneuverability to the locations described, either the messy dining table of drooling Empirico or the remoteness of the Corona Borealis supercluster.
Also, unfortunately, he did not tell you which beach or which hour the sand triangle would be drawn, nor in which of the countless galaxies of the supercluster the particular three stars and lines of nebula gas and dust the aliens used to describe the figure.
Also, Empirico makes no general statement about all right triangles, but only says that of the four of them, one has the Pythagorean property permanently, one temporarily and two others not at all.
So if you intend to build your house using a right triangle in the flying buttress, you will have no knowledge of whether it violates or abides by the Pythagorean property.
However, you also know that sometimes bridges fall over because of wind or gravity or rust or faulty load-bearing members, but you have never once heard tell of any bridge falling over because the triangular shapes of the braces lacked the Pythagorean property, whereas other triangular shaped braced had that Pythagorean property.
So here are my questions:
1. does the presence or absence of the Pythagorean property have any bearing whatsoever on how well or poorly bridges and houses stand? If so, what?
2. How is the equality of the area of a square built on the hypotenuse with squares built on the other two legs aid a flying buttress, for example, to buttress a wall?
3. Is Empirico more persuasive of the question under dispute than Pythagoras? In other words, if you were in the situation described, are you under obligation to believe Empiricio’s statements about his triangles of spit and sand and smoke and stars? Are you under any obligation to believe the conclusions of Pythagoras? (I phrase it this way because I am not concerned with your or any man’s personal desire to believe or disbelieve, only what you or he ought to believe.)
4. Did Empirico indeed prove his point that not all right triangles have the property that squares built on their hypotenuse equal the area of the squares built on the two remaining side?
5. If he did not prove his case, could Empirico have proven his case under other conditions?
6. What conditions?
7. What would he have to do to prove his case beyond reasonable doubt?
8. How many triangles would Empirico need to show you to prove the Pythagorean property were true of all triangles as opposed to merely some?
9. Did Pythagoras fail to prove his case?
10. If so, in what respect? Where is there a lapse or a leap in his logic?
11. If that lapse or leap in logic were corrected, or the general conclusion of his argument limited to a conditional category (such as, for example, by saying the Pythagorean theorem is true for a Euclidean right triangle but not true for any noneuclidean triangles, that is, triangles for whom we do not postulate that parallel lines neither diverge nor converge), would the case of Pythagoras be proved?
About John C Wright
John C. Wright is a practicing philosopher, a retired attorney, newspaperman, and newspaper editor, and a published author of science fiction. Once a Houyhnhnm, he was expelled from the august ranks of purely rational beings when he fell in love; but retains an honorary title.
